Question 4
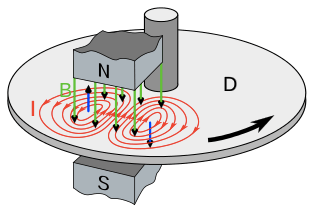
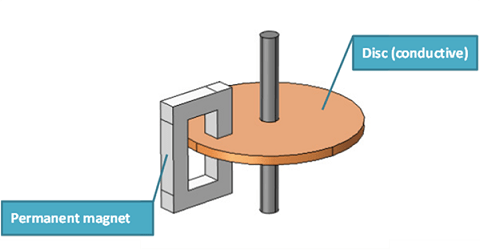
Eddy current brake

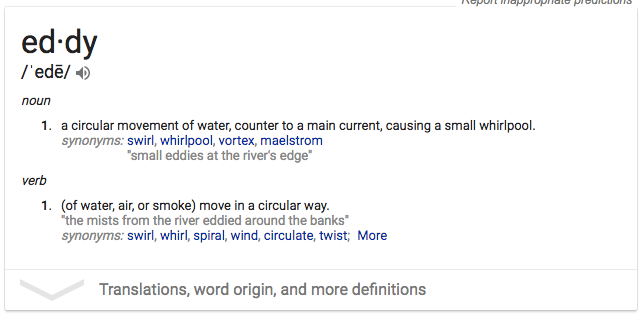
Eddy
Question 9
Magnetic Field due to a Current Loop
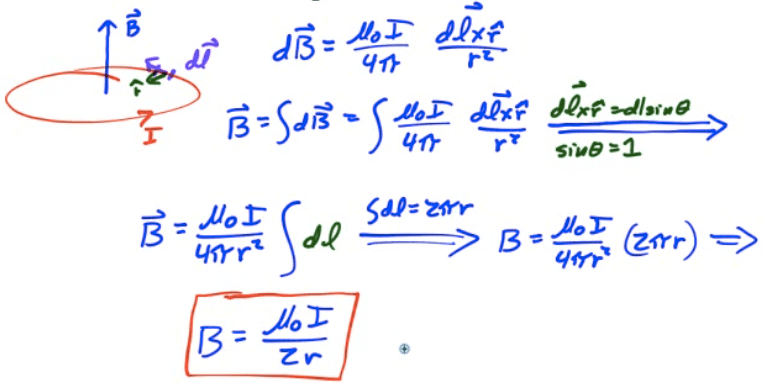
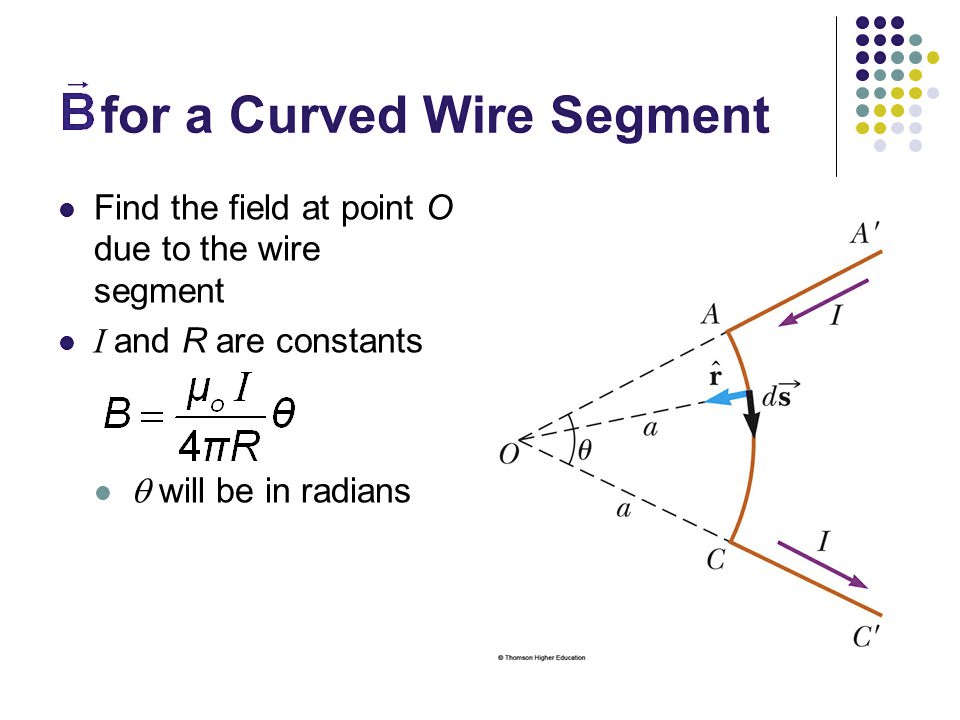
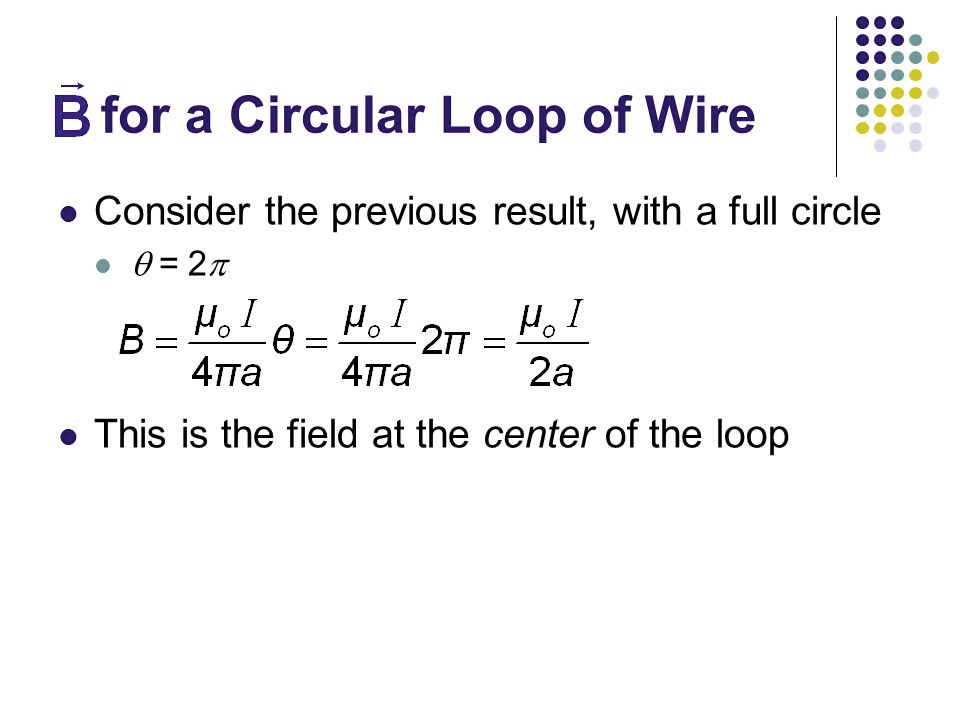
Question 10
Energy in a capacitor
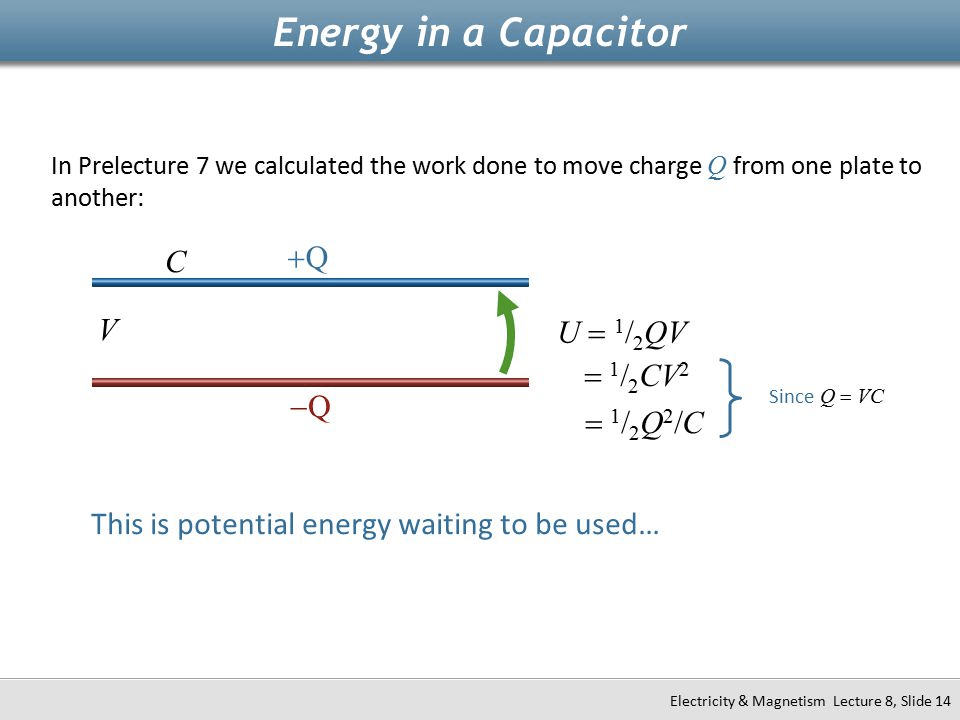
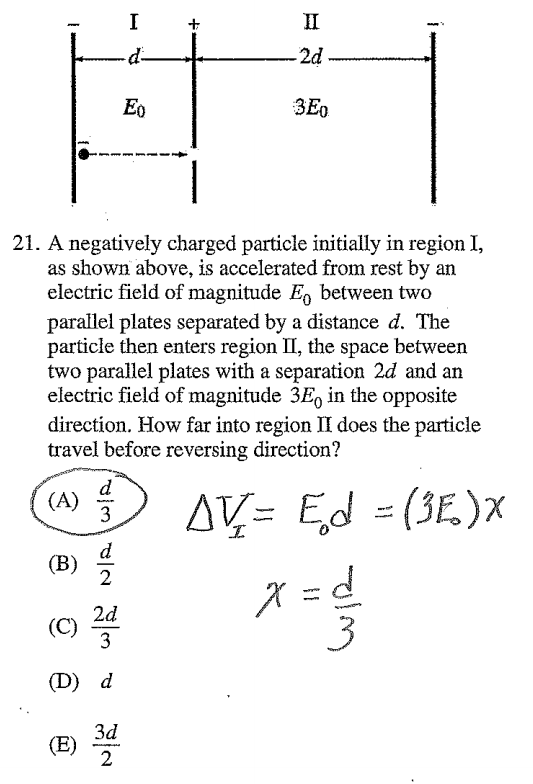
Question 21
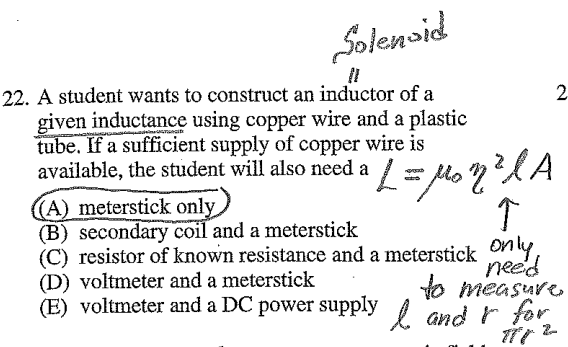
Question 22
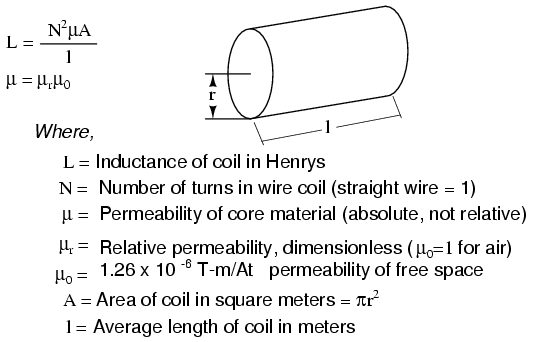
Formula for inductance of simple solenoids
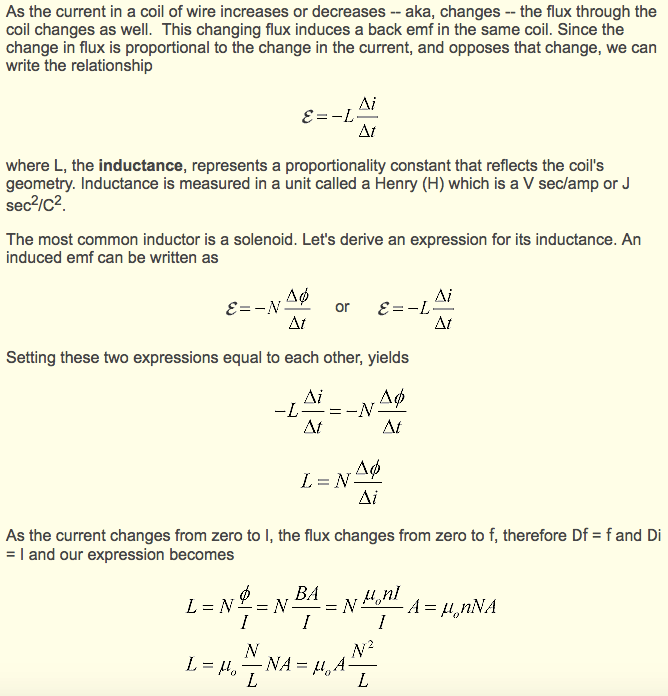
Derivation
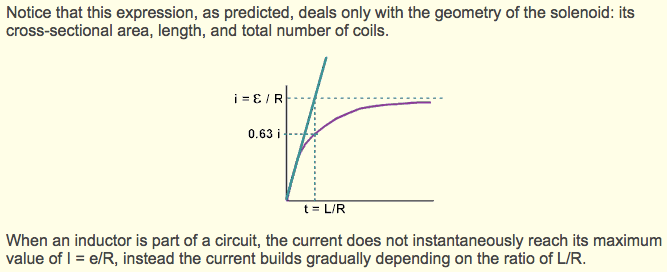
Question 23

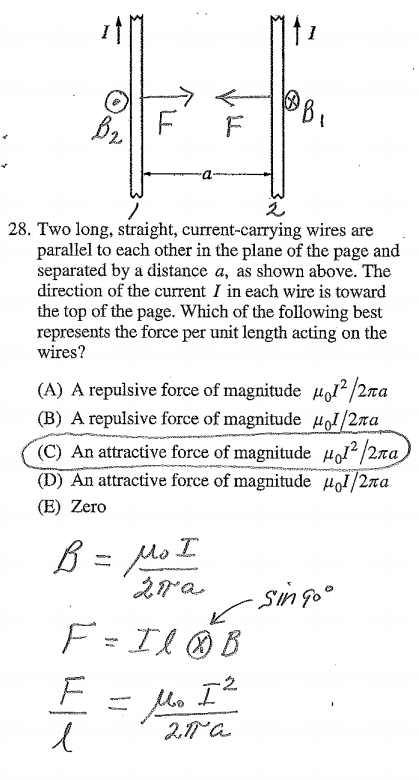
Question 28
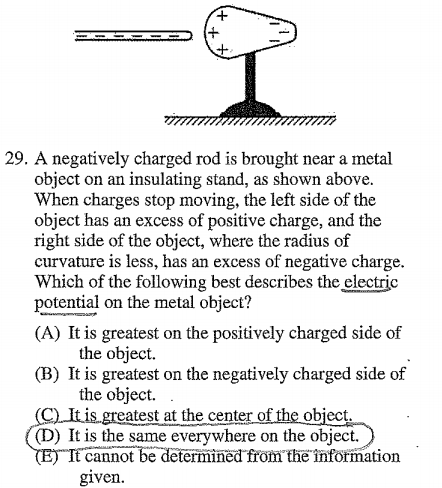
Question 29
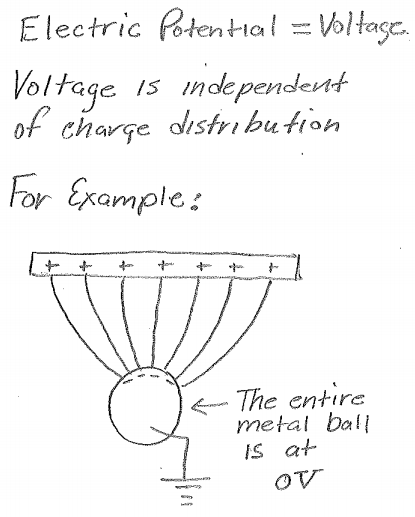
Question 30
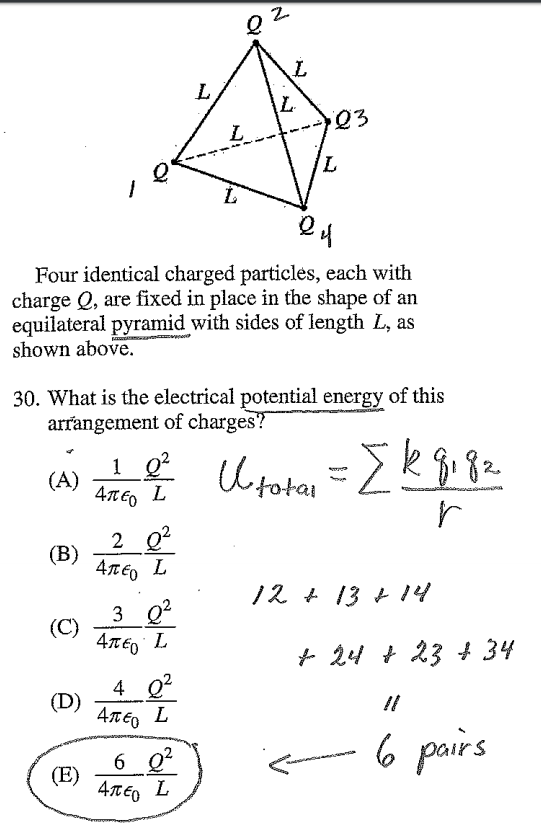
Question 31
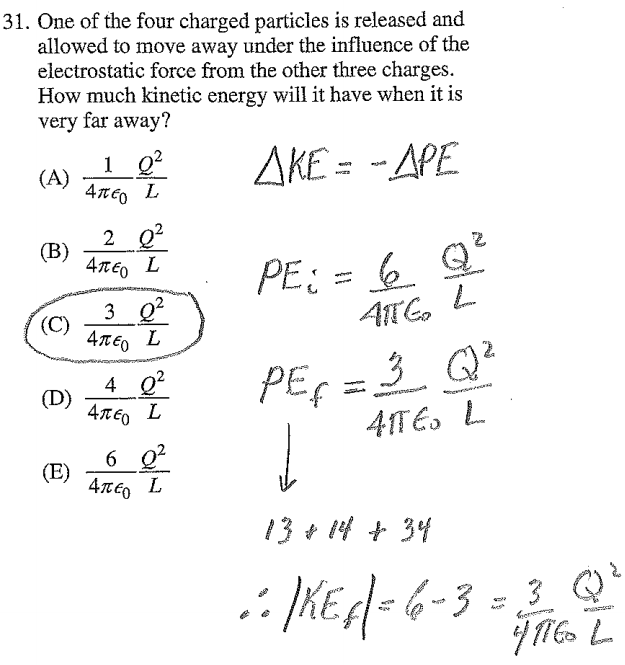
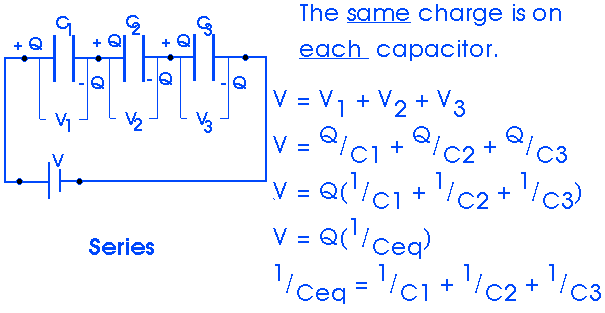
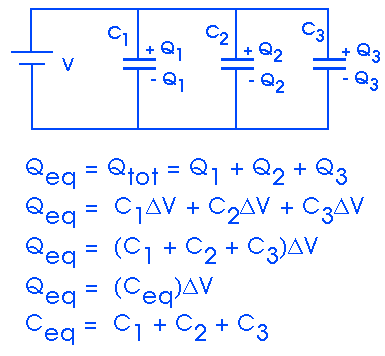
Question 33
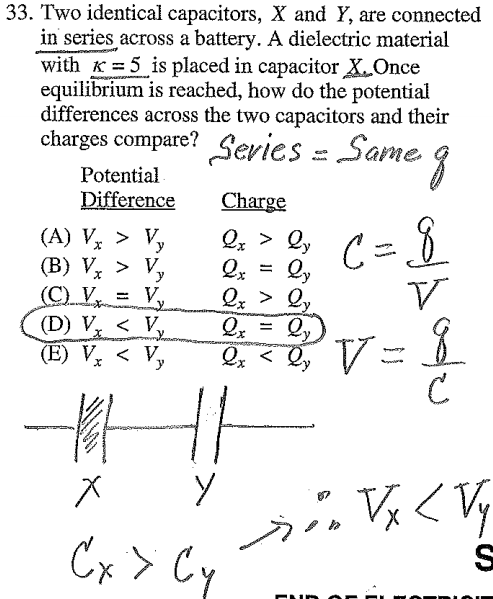
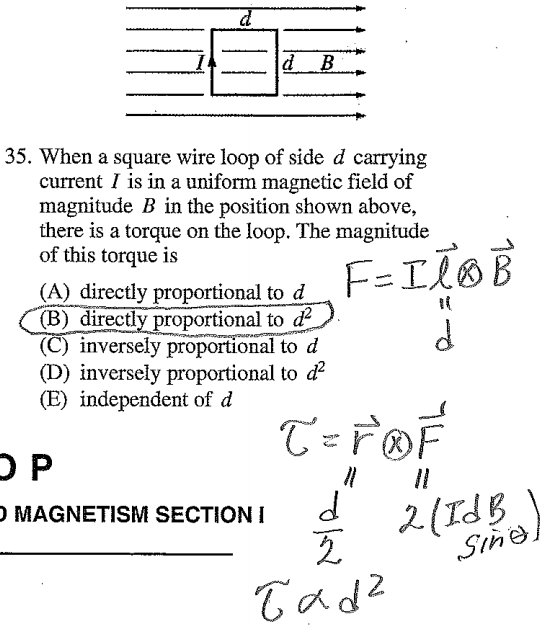
Question 35